So what exactly are perfect intervals? What makes them so special to be called “perfect”? An interval is an interval, right?
Before we get into the deep logistics of perfect intervals, let’s go through a quick review of what an interval in music is first.
Fast Interval Review
In music theory, an interval is described as the distance between two notes. Basically this is describing in greater detail how close or far apart two notes are from each other.
To define an interval, they are called unison, 2nd, 3rd, 4th, 5th, 6th, 7th or 8th (octave).
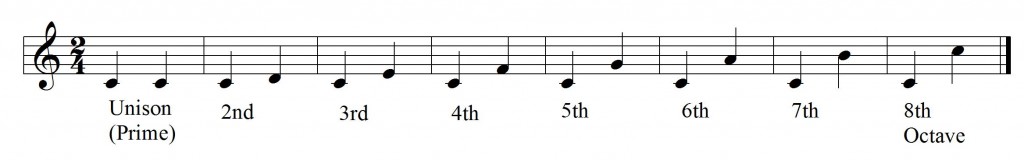

What is a Perfect Interval?
A perfect interval identifies the distance between the first note of a major scale and the unison, 4th, 5th or octave. Only those intervals can be given the extra attached name as “perfect”.


PU/PP/P1 = Perfect Unison/Perfect Prime
P4 = Perfect Fourth
P5 = Perfect Fifth
P8 = Perfect Octave
In the example listed above, all of the perfect intervals are found within the C Major scale.
Why is it Called Perfect?
That is a very good question that involves more historical analysis into the times of antiquity in order to really answer it properly. From my understanding, the term “perfect” originated due to the musical overtone series.
Apparently perfect intervals ring in a way that other intervals do not. These sound qualities were first discovered and praised in the East.
A guy named Pythagoras was the first person from the West to explore this interesting observation. He is responsible for incorporating it into our current musical language and practice.
The label of “perfect” in addition to a number describes the interval’s quality. These intervals are called perfect because the ratios of their frequencies are simple whole numbers.
Another Tool to Use
If one of the interval notes does not include the first note of a scale, it can be a little trickier to find out what the quality of the interval is. Just because the first note of any scale is not included does not mean it could not still be a perfect interval.
The way to find out is to count the number of half steps between the notes.
P1 = 0 half steps
P4 = 5 half steps
P5 = 7 half steps
P8 = 12 half steps
Start with the lower note and count in half steps moving up until you reach the last note. This should help you decide if the interval truly is “perfect”.
Here are some examples of perfect intervals that do not include the first note of the C Major Scale as the bottom note even though they are all still found with the same scale:

A perfect unison is very easy to find because both notes are exactly the same. Likewise, a perfect octave is also simple to detect. Both notes are the same except one of the notes are an octave (8 notes) higher or lower than the other note.
In Summary
1) Perfect intervals include adding a note above the first note of a major scale that represents the distance of a unison (prime), 4th, 5th or 8th (octave) interval.
2) A perfect interval does not have to include the first note of the major scale. As long as both notes are found in the same major scale, they are considered diatonic intervals. Using half steps as your guide will really help you determine what quality of intervals they are.
3) They are labeled as “perfect” because the sound quality is much different from any other intervals.
That is really all you need to know about perfect intervals! The more you understand the language of music, the better you get at reading and playing!
Music Interval Practice Resources
-
 $28.01Buy Now
$28.01Buy Now30 self-guided lessons and ready-to-use reproducible activity sheets for mastering intervals in music. They are ideal for choir, band, general music class, private lessons, and orchestra. Learn to recognize intervals through both sight and sound.
We earn a commission if you make a purchase through our links, at no additional cost to you.
02/18/2024 05:57 am GMT -
 $7.99Buy Now
$7.99Buy NowLearn music intervals in a fun and engaging way through these coloring pages. After identifying and coloring each interval, a unique geometric design appears.
We earn a commission if you make a purchase through our links, at no additional cost to you.
02/18/2024 06:45 pm GMT -
 $7.95Buy Now
$7.95Buy NowUse these flash cards to practice learning and memorizing music intervals on the grand staff. It includes intervals of a 2nd through an octave. They are best for piano students.
We earn a commission if you make a purchase through our links, at no additional cost to you.
02/18/2024 04:22 pm GMT
Helpful Music Theory Resources
This foldable 8x10 inch music theory cheat sheet is an excellent quick reference guide when you need to find the answer fast. The side 3-hole punch allows you to keep it in a 3-ring binder. It is sturdy and folds out featuring music theory and notation on the front and music history on the back.
A practical pocket-size music theory dictionary and music notation reference guide that is perfect for all musicians from beginner to professional.
A convenient music theory book that is small enough to fit in your pocket, backpack, or instrument case. A great reference guide for all musicians at any level of music study.



Thank you so much for such an informative little article. The question came up in our college band “What makes a perfect interval perfect?” You’re article was spot on, thanks!
So glad to hear this was helpful! Thanks for all the kind words and feedback 🙂
Hi Teresa, I love your website. This is really useful. Thank you!
Thank you for a concise explanation of the perfect interval in your article. The topic came up in a piano lesson with a student
I really like this website for the demystifying and easy to follow explanations.
I was intrigued by these ‘perfect’ intervals and, being a boring accountant, looked at the maths of the tone vibrations. The perfect octave of concert A (440Hz) rings at 880Hz – exactly two vibrations for every one vibration of the root tone. The perfect fourth rings at very close to 587Hz – one and one third vibrations for every one of the root tone. And the perfect fifth rings at very close to 660Hz – one and one half vibrations for every one of the root tone. I think when our ears hear these perfect intervals our brains neatly slot the faster vibrations in between the slower ones, tidily filling up the gaps, like completing a jigsaw. None of the other intervals have anywhere near such tidy ratios to the root tone, so they jar to some degree, like a jigsaw with missing (or extra) pieces.
Mathematically this all makes ‘perfect’ sense to me. Still battling with the piano though!
Thanks for this great musical resource.
You say “To define an interval, they are called unison, 2nd, 3rd, 4th, 5th, 6th, or 8th (octave).” but you show a staff that contains a 7th. What happened to the 7th in the sentence I quoted?
Thank you. I have made the correction.
U are simply genius the way u explained it was very different..i read about intervals but never without something not fully understood..this answered my questions perfectly..Thank you..
Thank you for the eloquent explanation, I had big headache understanding what P means,
Bookmarked! Thank you for this simple explanation. I couldn’t proceed forward without knowing WHY? Why is it perfect? Demystified it for me you did. 🙂
Kat, I’ve been straining my brain over this for the last several days. I’m an engineer by training, and really like for things to be rigorous. I’ve seen the definition that an interval is perfect if it inverts to a perfect interval. But that seems to have a bootstrapping problem. We say that (in the C major scale) C-G is a fifth, and it inverts to a fourth. And since the fourth is perfect, then the fifth is. But why is the fourth perfect? Why, because the fifth is, by the same reasoning. See the problem?
I’ve been hacking on the scales myself to try to find something more solid, and I did notice this: in the C major scale, the note G is seven semitones above the root. Five additional semitones are required to cover an octave, and the C major scale also contains a note five semitones above the octave: F (the 4th). No other notes in the octave have their “matching to cover an octave” note also in the scale. So the 4th and 5th stand out as unique in that way.
This turns out to be mode dependent, however. The C major scale is the Ionian mode. Other modes present us with different sets of notes that have this characteristic. Listing the modes in circle of fifth order, I came up with the following:
Lydian: 4th only (it’s six semitones above the root – it’s its own partner)
Ionian: 4th+5th
Mixolydian: 4th+5th, 2nd+7th
—————————————- major modes above, minor modes below
Dorian: all notes
Aeolian: 4th+5th, 2nd+7th
Phrygian: 4th+5th
Locrian: 5th only (six semitones – its own partner again)
I find the pattern exhibited here fascinating, and it seems like it must be telling us something. But I’m not a musical professional – just a well-educated rational thinker that likes to dig for structure and pattern.
Do you see anything here that seems interesting?
I don’t know why I used “Kat” in the previous comment, Teresa. Please change that for me if you can.
Kip
I’ve spent years trying to find out why perfect intervals are “perfect;” you’ve finally given me the answer! Thanks!
So glad to help!
This is so explicit and very easy to understand. Nice Job.
I’m studying AMEB Music Theory by distance education in NSW, Australia. I’m finding the course quite accessible, but just sometimes, the lack of information, such as “perfect intervals” is a worry to me. Since reading your explanation, above, I can now comprehend the term, “Perfect”. I went to my piano and tested your words, which are true. I have been a classical singer all my life and have relied on my ears very much, now I have the time to go more theoretical. I’m signing up. William Amer
Thankyou.
Why, oh why does nobody mention the hymn tune FOREST GREEN (used in England for O Little Town of Bethlehem) as an example of the perfect fourth interval?
Forest Green is the best melody ever composed.
Claire Dixon
aka “Forest Green Organ Geek”
What’s perfect about the perfect intervals?
If you look at the ratios of intervals in a major diatonic scale in just intonation, the perfect intervals are the only intervals whose inversions are also intervals in the major diatonic scale.
1:1, 9:8, 5:4, 4:3, 3:2, 5:3, 15:8, 2:1
1:1 > 1:1______________YES
9:8 > 8:9 > 16:9________NO
5:4 > 4:5 > 8:5_________NO
4:3 > 3:4 > 6:4 = 3:2_____YES
3:2 > 2:3 > 4:3_________YES
5:3 > 3:5 > 6:5_________NO
15:8 > 8:15 > 16:15_____NO
2:1 > 1:2 > 2:2 = 1:1____YES
Please explain, with an example, what you mean by: “These intervals are called perfect because the ratios of their frequencies are simple whole numbers.” I tried working some math and couldn’t come up with a whole number.
Thanks,
Larry Linne
[email protected]
On February 21, 2015 at 1:23, above, Rowan Hill says:
“I was intrigued by these ‘perfect’ intervals and, being a boring accountant, looked at the maths of the tone vibrations. The perfect octave of concert A (440Hz) rings at 880Hz – exactly two vibrations for every one vibration of the root tone…” Why did he use “concert A (440Hz)” as the root tone?
Thanks,
Larry Linne
Thank you for putting your time into this!
I hope not to confuse this, but there is another reason they are called perfect.
When inverting intervals, minor intervals become major, diminished intervals become augmented, but perfect intervals become … PERFECT!
That is to say perfect intervals are the only intervals that do not change quality when inverted. They are therefore perfect intervals!
Did not clear up a thing. If they are arbitrarily defined as perfect that’s fine. But this article did nothing to explain why.
I’m sorry, but after reading the article, I still had no idea why these intervals are called perfect. For example, saying “These intervals are called perfect because the ratios of their frequencies are simple whole numbers,” means nothing to me. Perhaps if it was explained what the ratios of frequencies are, with some examples thrown in.
I did like Peter’s comment of September 3, that “…perfect intervals are the only intervals that do not change quality when inverted…”
That makes some sense to me.
But the simplest explanation I’ve seen so far, and my favorite, I found on another website:
“Perfect intervals are the ones that don’t have two forms: major and minor.”
That makes sense to me, and here’s why:
One of the definitions of the word “perfect” is: “precisely accurate; exact,” as in “a perfect circle.”
A fifth, for example, is an exact, precise interval. It needs no further adjective, like major or minor, to describe it.
Would it be safe to say that those intervals are called perfect because whether you are in a major or minor scale the note is the same?
A A
B B
C# C
D perfect D
E perfect E
F# F
G# G
A perfect A